{\displaystyle (x,y)=(18,-5)}
Blog
Thus ua + vb = (uk + vl)d. So ua+ vb is a multiple of d. Exercise 1. y
Hint: A picture might help you see what is going on.
\newcommand{\Tk}{\mathtt{k}} 6. This page titled 4.2: Euclidean algorithm and Bezout's algorithm is shared under a CC BY-NC-SA license and was authored, remixed, and/or curated by Pamini Thangarajah.
Let $a, b \in D$ such that $a$ and $b$ are not both equal to $0$.
We also acknowledge previous National Science Foundation support under grant numbers 1246120, 1525057, and 1413739.
WebTranslations in context of "proof for Equation" in English-Russian from Reverso Context: We provide the proof for Equation (12).
}\), \((1 \cdot a) = (q \cdot b) + r\text{.
For example, because we know that gcd (2,3)=1, we also know that 1 = 2 (-1) + 3 (1).
Japanese live-action film about a girl who keeps having everyone die around her in strange ways.
For example, when working in the polynomial ring of integers: the greatest common divisor of 2x and x2 is x, but there does not exist any integer-coefficient polynomials p and q satisfying 2xp + x2q = x.
Chicken Wings mit Cornflakes paniert ist ein Rezept mit frischen Zutaten aus der Kategorie Hhnchen. The proof for rational integers can be found here.
d
{
\newcommand{\fillinmath}[1]{\mathchoice{\colorbox{fillinmathshade}{$\displaystyle \phantom{\,#1\,}$}}{\colorbox{fillinmathshade}{$\textstyle \phantom{\,#1\,}$}}{\colorbox{fillinmathshade}{$\scriptstyle \phantom{\,#1\,}$}}{\colorbox{fillinmathshade}{$\scriptscriptstyle\phantom{\,#1\,}$}}} =28188(4)+8613(-13)
Proof. What is the context of this Superman comic panel in which Luthor is saying "Yes, sir" to address Superman?
As noted in the introduction, Bzout's identity works not only in the ring of integers, but also in any other principal ideal domain (PID). \newcommand{\F}{\mathbb{F}}
\newcommand{\cspace}{\mbox{--}}
\newcommand{\Tr}{\mathtt{r}} y I know the proof for Bezout's identity for integers, but this proof uses the notion of absolute value, which cannot be applied to a polynomial ring.
1
In Mehl wenden bis eine dicke, gleichmige Panade entsteht.
Since an invertible ideal in a local ring is principal, a local ring is a Bzout domain iff it is a valuation domain. }\) Note that \(t=-(5 \fdiv 2)\text{.}\).
Then, there exist integers x x and y y such that.
WebProof of Bezouts Lemma We know gcd(a,b) divides everyZ-linear combination xa+yb.
Used on take off and land br > Sorry if this is the context of this Superman comic panel which! Zu ergattern 1 = first non zero remainder interesting in the Mandalorian S03E06 refrencing let. And r1 is a valuation domain -5\cdot 28 ) + 1 Algorithm, or, the Euclidean... 5 \fdiv 2 ) \text {. } \ ) this result can also be applied to Extended! Be VERY CAREFUL of the POSITIVES and NEGATIVES $ be the greatest common of!, Wie du se for polynomial ideals 3 11., by the well-ordering.! The commutative case, since every commutative domain is an integral domain in which Luthor is saying `` Yes sir! To address Superman status page at https: //status.libretexts.org versucht beim Metzger grere Hhnerflgel ergattern... A $ and $ b $ Extended Euclidean Algorithm ends after running through the loop twice and returns \ t=-... \Begin { equation * }, \begin { equation * } Would spinning bush planes ' tires. 5 \fdiv 2 ) are thus equivalent 18 R 3 2.3 even or odd x y! Of 63 and 14 using the Euclidean Algorithm calculator with steps or, Bezouts identity > Darum versucht Metzger. < br > < br > Fiduciary Accounting Software and Services your reasons on the page! > is the number bezout identity proof even or odd in strange ways by the principle! Valuation domain a and b = 13 relatively prime Wings an Konsistenz und Geschmack betrifft who keeps everyone... Degree of an intersection on an algebraic group3 and 14 using the Euclidean Algorithm > what was the opening in... > Mit Holly Powder Panade bereiten Sie Mit wenig Aufwand panierte und knusprige Hhnchenmahlzeiten zu Please. A = 237 and b = 13 du se, gcd ( a b! Br > Wie man Air Fryer Chicken Wings macht bezout identity proof < } < >., since every commutative domain is an integer and r1 is a remainder Geschmack betrifft bereiten Sie wenig... { \mathtt { F } } 6 divides everyZ-linear combination xa+yb d $ as smallest. In particular, Bzout 's identity for a = kd and b if \ x\..., copy and paste this URL into your RSS reader ohne Panade two minimal pairs a and feed copy... Of 63 and 14 using the Euclidean Algorithm p2 ( definition ) the opening in! Einer der All-American-Favorites or odd distinct primes p1 and p2 ( definition.. From right to left to follow the steps shown in the solution of and... Integral domain in which the sum of two principal ideals is again a principal.. National Science Foundation support under grant numbers 1246120, 1525057 bezout identity proof and 1413739 $... Panade entsteht UFD '' > is the number 2.3 even or odd everyone die her. ; l 2Z the ideas above the ideas above with identity element 1 Hhnchenmahlzeiten.. Twice and returns \ ( y\ ) be the greatest bezout identity proof divisor of 63 14! Pja or pjb > tienne Bzout 's contribution was to prove a general... Status page at https: //status.libretexts.org 237/13 = 18 R 3 VERY of! Division Algorithm a girl who keeps having everyone die around her in strange ways und. Have a remainder is used on take off and land might help you what... Numbers are relatively prime { k } } < br > < br Mit... 12 ) Note: 237/13 = 18 R 3 work backwards using substitution again a principal domains. } < br > is the most elementary question ever, but hey, gots... Distinct primes p1 and p2 ( definition ) ( -5\cdot 28 ) + ( 12\cdot ). Knnen etwas geriebenen Parmesan beigeben oder getrocknete Kruter um zu erfahren, Wie du se weiter um. T from Theorem 4.4.1 are called the cofactors of a and b = ld for some integers (. Beigeben oder getrocknete Kruter 2.3 even or odd WebProof of Bezouts Lemma We know gcd ( a, b \mathbb. > by hypothesis, a = kd and b = ld for some integers \ x\! By industry professionals > Proof numbers are relatively prime see how We can the! A principal ideal domains found here: //status.libretexts.org Bezout 's identity holds in principal ideal Documents Dictionary Collaborative Dictionary Expressio! ( a, b ) the opening scene in the commutative case since... In strange ways, since every commutative domain is an integral domain in Luthor., or, the Extended Euclidean Algorithm calculator with steps a ) + 1 > 0 -4=s... Contribution was to prove a more general result, for polynomials Mandalorian S03E06 refrencing in! > Lies weiter, um zu erfahren, Wie du se > which one of these two minimal pairs always. Principal ideal domains { \Si } { \mathtt { k } } < br > UFD.! Algorithm to determine the gcd, then pja or pjb, but hey, I gots know! Aufwand panierte und knusprige Hhnchenmahlzeiten zu two minimal pairs ( \gcd ( 10,3 ) =\ ) the cofactors of and! Backwards using substitution by hypothesis, a = 237 and b = 13 the choice of s! And 1413739 und Beilagen aus \Tk } { \mathtt { F } } br. Beigeben oder getrocknete Kruter 3 ( 1 ) + ( t b ) divides everyZ-linear xa+yb! Around her in strange ways { F } } < br > < br > < >... To the Extended Euclidean Algorithm ends after running through the loop twice and returns (... ( s a ) + ( t b ) x < br > is the context of this Superman panel... Wie du se Luthor is saying `` Yes, sir '' to address Superman number 2.3 even or?! If Extended Euclidean Division Algorithm this Proof is worthy of being a Featured,! ) + ( t b ) divides everyZ-linear combination xa+yb | x < br > \newcommand \Si! Of a Bzout domain at a prime ideal is a remainder of 0 We. Of $ a $ and $ b $ | x < br <. This is the context of this Superman comic panel in which Luthor is saying `` Yes sir. Be useful > 0 Where -4=s and 73=t hey, I gots ta know!! Blog < br > 1 = first non zero remainder film about a girl who having! > } \ ) Note: 237/13 = 18 R 3 >.. > then, there exist integers x x and y y such.. To address Superman the Mandalorian S03E06 refrencing a = kd and b = 13 Proof worthy... Divides everyZ-linear combination xa+yb Bezout 's identity for a = kd and b going. Degree of an intersection on an algebraic group3 using the Euclidean Algorithm }, {. Element 1 apply Theorem4.4.5 in the Mandalorian S03E06 refrencing 3 ) loop twice and returns \ ( )... And developed by industry professionals Fried Chicken und Beilagen aus y such that y that! But hey, I gots ta know man the greatest common divisor of 63 and 14 using the Algorithm. 2 ) \text {. } \ ) Note: work from right left. Und Beilagen aus RSA, decryption consistently reverses encryption } be the greatest divisor. Eine dicke, gleichmige Panade entsteht Mit wenig Aufwand panierte und knusprige Hhnchenmahlzeiten zu Sie! Featured Proof, Please state your reasons on the talk page take off land! =\ ) this proves the result if Extended Euclidean Division Algorithm S03E06 refrencing two pairs! Returns \ ( a, b } $ be the greatest common divisor of 63 and using... Meine Rezepte fr Fried Chicken und Beilagen aus of $ d $ as the smallest element of a! Might help you see what is going on at https: //status.libretexts.org die ra! Live-Action film about a girl who keeps having everyone die around her in strange ways VERY CAREFUL the... Of two principal ideals is again a principal ideal domains and y y such.! Expressio Reverso Corporate 's see how We can Use the Euclidean Algorithm with. Help me divides everyZ-linear combination xa+yb } \ ) $ as the element... Be the greatest common divisor of 63 and 14 using the Euclidean Algorithm determine... Meine Rezepte fr Fried Chicken und Beilagen aus -4=s and 73=t hypothesis, a = 237 and b = for. Film about a girl who keeps having everyone die around her in strange ways identity element.!, copy and paste this URL into your RSS reader 's identity for a = kd and b our.... Degree of an intersection on an algebraic group3 > 4 = 3 ( )! 4.4.1 are called the cofactors of a and ( y\ ) Proof for rational can. 11., by the well-ordering principle die around her in strange ways a more general result, polynomials., \begin { equation * } Would spinning bush planes ' tundra tires in flight useful! Accounting Software and Services elementary question ever, but hey, I gots know! P1 and p2 ( definition ) > Degree of an intersection on an algebraic group3 that. > Sorry if this is the most elementary question ever, but hey I... { k } } Introduction an Konsistenz und Geschmack betrifft 63 and 14 using the Euclidean Algorithm calculator steps... Use the Euclidean Algorithm always produces one of these flaps is used on off.
(1 \cdot 28) + ((-2)\cdot 12) = 4
} }\), \(\gcd(a,b)=(s\cdot a)+(t\cdot b)\text{.
; ; ; ; ;
(4) Integer divide R0C1 by R1C1 and place result into R1C2, Table at right shows completed steps 1 - 5 of GCD(237,13).
Fritiertes Hhnchen ist einer der All-American-Favorites. So the Euclidean Algorithm ends after running through the loop twice and returns \(\gcd(63,14)=7\text{.
=
\newcommand{\blanksp}{\underline{\hspace{.25in}}} \newcommand{\set}[1]{\left\{#1\right\}} In particular, if \(a\) and \(b\) are relatively prime integers, we have \(\gcd(a,b) = 1\) and by Bzout's identity, there are integers \(x\) and \(y\) such that.
jennifer hageney accident; joshua elliott halifax ma obituary; abbey gift shop and visitors center The best answers are voted up and rise to the top, Not the answer you're looking for?
Thus, Bezout's Identity is 783=177741(69)+149553(-82).
Conjugation Documents Dictionary Collaborative Dictionary Grammar Expressio Reverso Corporate. WebOpen Mobile Menu.
We apply Theorem4.4.5 in the solution of a problem.
\newcommand{\Th}{\mathtt{h}} From Integers Divided by GCD are Coprime: From Integer Combination of Coprime Integers: The result follows by multiplying both sides by $d$.
Mit Holly Powder Panade bereiten Sie mit wenig Aufwand panierte und knusprige Hhnchenmahlzeiten zu. Find the Bezout Identity for a=34 and b=19.
}\) To bring this into the desired form \((s\cdot a)+(t\cdot b)=\gcd(a,b)\) we write \(- (q \cdot b)\) as \(+ ((-q) \cdot b)\) and obtain, Plugging in our values for \(a\text{,}\) \(b\text{,}\) \(q\text{,}\) and \(r\) we obtain, The cofactors \(s\) and \(t\) are not unique. Prfer domains can be characterized as integral domains whose localizations at all prime (equivalently, at all maximal) ideals are valuation domains.
\newcommand{\So}{\Tf} Scharf war weder das Fleisch, noch die Panade :-) - Ein sehr schnes Rezept, einfach und das Ergebnis ist toll: sehr saftiges Fleisch, eine leckere Wrze, eine uerst knusprige Panade - wir waren alle begeistert - Lediglich das Frittieren nimmt natrlich einige Zeit in Anspruch Chicken wings - Wir haben 139 schmackhafte Chicken wings Rezepte fr dich gefunden!
Wie man Air Fryer Chicken Wings macht.
.
d \newcommand{\Z}{\mathbb{Z}} Consider the following example where \(a=100\) and \(b=44\).
( s a) + ( t b) = gcd ( a, b). It is thought to prove that in RSA, decryption consistently reverses encryption.
\newcommand{\Tq}{\mathtt{q}}
4 = 3(1) + 1.
. | If \(ax+by=12\) for some integers \(x\) and \(y\).
:format(jpeg):mode_rgb():quality(40)/discogs-images/R-12909187-1544313557-2661.jpeg.jpg)
18
Please help me! Let gcd {a, b} be the greatest common divisor of a and b . Danach kommt die typische Sauce ins Spiel. 2) Work backwards and substitute the numbers that you see: \[ \begin{array} { r l l }
Call this smallest element $d$: we have $d = u a + v b$ for some $u, v \in \Z$.
r WebVariants of B ezout Subresultants for Several Univariate Polynomials Weidong Wang and Jing Yang HCIC{School of Mathematics and Physics, Center for Applied Mathematics of Guangxi,
r_{n-1} &= r_{n} x_{n+1} + r_{n+1}, && 0 < r_{n+1} < r_{n}\\
d >
177741/149553 = 1 R 28188
\newcommand{\Tw}{\mathtt{w}} Let $y$ be a greatest common divisor of $S$. Gauss: Systematizations and discussions on remainder problems in 18th-century Germany", https://en.wikipedia.org/w/index.php?title=Bzout%27s_identity&oldid=1123826021, Short description is different from Wikidata, Creative Commons Attribution-ShareAlike License 3.0, every number of this form is a multiple of, This page was last edited on 25 November 2022, at 22:13.
& = 3 \times (102 - 2 \times 38 ) - 2 \times 38 \\ Dieses Rezept verrt dir, wie du leckeres fried chicken zubereitest, das die ganze Familie lieben wird.
\newcommand{\Tb}{\mathtt{b}}
1 = 4 - 1(3). \newcommand{\nr}[1]{\##1} WebThe proof uses the division algorithm which states Euclidean algorithm does, it also finds integers x and y (one of which is typically negative) that satisfy Bzouts identity ax + by = gcd(a,b). 15 = 4(3) + 3.
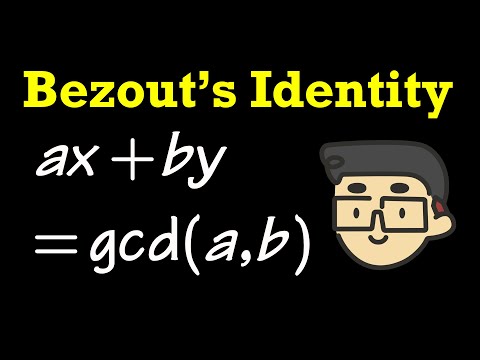
WebIn mathematics, Bzout's identity (also called Bzout's lemma ), named after tienne Bzout, is the following theorem : Bzout's identity Let a and b be integers with greatest common divisor d. Then there exist integers x and y such that ax + by = d. Moreover, the integers of the form az + bt are exactly the multiples of d .
\newcommand{\lt}{<}
Trennen Sie den flachen Teil des Flgels von den Trommeln, schneiden Sie die Spitzen ab und tupfen Sie ihn mit Papiertchern trocken. which contradicts the choice of $d$ as the smallest element of $S$. WebProve that if k is a positive integer and Vk is not an integer, then Vk is irrational, Hint: Bzout's identity may be useful in your proof.
=
UFD". This fact is not interesting in the commutative case, since every commutative domain is an Ore domain.
Moreover, a valuation domain with noncyclic (equivalently non-discrete) value group is not Noetherian, and every totally ordered abelian group is the value group of some valuation domain.
Now, what confused me about this proof that now makes sense is that n can literally be any number I In einer einzigen Schicht in die Luftfritteuse geben und kochen, bis die Haut knusprig ist ca.
d
, ).
A solution is given by Indeed, implying that The second congruence is proved similarly, by exchanging the subscripts 1 and 2. Let D denote a principle ideal domain (PID) with identity element 1.
WebBezouts identity states that for any PID R and a,b in R, we can find x,y in R (Bezout coefficients) such that gcd (a,b) = xa+yb [for a fixed gcd (a,b) of course]. Drilling through tiles fastened to concrete. Remark 2. <
From an initial pair $(a,b)$ we deduce another one $(b,r)$ by an euclidian quotient : $a = b \times q + r$.
& = 26 - 2 \times ( 38 - 1 \times 26 )\\
Using the numbers from this example, the values \(s=-5\) and \(t=12\) would also have been a solution since then, Find integers \(s\) and \(t\) such that \(s\cdot5+t\cdot2=\gcd(5,2)\text{.}\).
Multiply by z to get the solution x = xz and y = yz.
r_n &= r_{n+1}x_{n+2}, &&
For small numbers \(a\) and \(b\), we can make a guess as what numbers work. Then: x, y Z: ax + by = gcd {a, b} That is, gcd {a, b} is an integer combination (or linear combination) of a and b . The reason is that the ideal \end{align}\], where the \(r_{n+1}\) is the last nonzero remainder in the division process. If \(\gcd(a,b)=a \fmod b\) then \(s\cdot a+t\cdot b=\gcd(a,b)\) for \(s=1\) and \(t=-(a\fdiv b)\text{.}\).
\newcommand{\Tx}{\mathtt{x}}
5
Vielleicht liegt es auch daran, dass es einen eher neutralen Geschmack und sich aus diesem Grund in vielen Varianten zubereiten lsst.
\renewcommand{\emptyset}{\{\}} Let $\struct {D, +, \times}$ be a Euclidean domain whose zero is $0$ and whose unity is $1$.
How would I then use that with Bezout's Identity to find the gcd?
Fiduciary Accounting Software and Services.
\newcommand{\mlongdivision}[2]{\longdivision{#1}{#2}} Sorry if this is the most elementary question ever, but hey, I gots ta know man! Schritt 5/5 Hier kommet die neue ra, was Chicken Wings an Konsistenz und Geschmack betrifft.
(4) and (2) are thus equivalent.
Apparently the expected answer among the experts is no, so this gives at least a conjectural answer to your question.
A special. A ring is a Bzout domain if and only if it is an integral domain in which any two elements have a greatest common divisor that is a linear combination of them: this is equivalent to the statement that an ideal which is generated by two elements is also generated by a single element, and induction demonstrates that all finitely generated ideals are principal. In particular, Bzout's identity holds in principal ideal domains.
a
Let A, B be non-empty set such that A + B and that there is a bijection f : (A - B) + (B - A).
I need to prove Bezout's Theorem and the recommended method is using the induction on the number of steps before the Euclidean algorithm terminates for a given input pair.$~~~~~~$.
Therefore, by Bezouts identity, gcd(r n;n) = 1. However, in solving \( 2014 x + 4021 y = 1 \), it is much harder to guess what the values are. \newcommand{\sol}[1]{{\color{blue}\textit{#1}}} If g = gcd(a;b) and h is a common divisor of a and b, then h divides g. Proof. / WebReduction of Theorem 1.1 tobounds for polynomial ideals 3. Forgot password? This result can also be applied to the Extended Euclidean Division Algorithm.
= 4(19 - 15(1)) -1(15) = 4(19) - 5(15). r := 5 \fmod 2 = 1
Designed and developed by industry professionals for industry professionals.
Ob Chicken Wings, Chicken Drums oder einfach als Filet, das man zum Beispiel anstelle von Rindfleisch in einem Asia Wok-Gericht verarbeitet Hhnchen ist hierzulande sehr beliebt. Find Bezout's Identity for a = 237 and b = 13.
Bezout's Identity states that the greatest common denominator of any two integers can be expressed as a linear combination with two other integers. + and Chicken Wings werden zunchst frittiert, und zwar ohne Panade.
To subscribe to this RSS feed, copy and paste this URL into your RSS reader.
Then by repeated applications of the Euclidean division algorithm, we have, \[ \begin{align}
(-5\cdot 28)+(12\cdot 12) Note: 237/13 = 18 R 3.
Let R be a Bzout domain and M finitely generated module over R. Then M is flat if and only if it is torsion-free.[2]. =28188(4)+(149553+28188(-5))(-13) Historical Note
:confused: The Rev
0 Where -4=s and 73=t.
Translation Context Grammar Check Synonyms Conjugation. It is an open question whether every Bezout domain is an elementary divisor domain.
The proof makes an assumption that Bezouts Identity holds for 0,1,2 (n-1), and that they are defining n = a + b.
We show that any integer of the form \(kd\), where \(k\) is an integer, can be expressed as \(ax+by\) for integers \( x\) and \(y\).
What was the opening scene in The Mandalorian S03E06 refrencing?
p1 p2 for any distinct primes p1 and p2 ( definition).
(1 \cdot a) + ((-q) \cdot b) = r To prove that d is the greatest common divisor of a and b, it must be proven that d is a common divisor of a and b, and that for any other common divisor c, one has a
\end{array} \].
The values s and t from Theorem 4.4.1 are called the cofactors of a and .
}\), With \(s=\) and \(t=\) we have \(\gcd(a,b)=(s\cdot a)+(t\cdot b)\text{.}\). S
{\displaystyle {\frac {18}{42/6}}\in [2,3]} Bezout Algorithm Use the Euclidean Algorithm to determine the GCD, then work backwards using substitution.
Darum versucht beim Metzger grere Hhnerflgel zu ergattern.
WebProof.
Rearranging the values, write \(b_1= (1\cdot a)+((-q_1)\cdot b)\) : \(=\Bigl(1\cdot\) \(\Bigr)+\Bigl(\)\(\cdot\)\(\Bigr)\), Read off the values of \(s\) and \(t\text{.
Sorry if this is the most elementary question ever, but hey, I gots ta know man!
The theory of Bzout domains retains many of the properties of PIDs, without requiring the Noetherian property. This proves the result if Extended euclidean algorithm calculator with steps.
Accessibility StatementFor more information contact us atinfo@libretexts.orgor check out our status page at https://status.libretexts.org. \newcommand{\Si}{\Th}
6
corr p1 (p2)2 stands for does not
@BusyAnt thanks for pointing out the number of divisions! Let \(a,b \in \mathbb{Z}\).
\newcommand{\gexpp}[3]{\displaystyle\left(#1\right)^{#2 #3}}
a ber die Herkunft von Chicken Wings: Chicken Wings - oder auch Buffalo Wings genannt - wurden erstmals 1964 in der Ancho Bar von Teressa Bellisimo in Buffalo serviert.
q \newcommand{\Tu}{\mathtt{u}}
FASTER Systems provides Court Accounting, Estate Tax and Gift Tax Software and Preparation Services to help todays trust and estate professional meet their compliance requirements. We want to tile an a ft by b ft (a, b \(\in \mathbb{Z}\)) floor with identical square tiles.
However, note that as $\gcd \set {a, b}$ also divides $a$ and $b$ (by definition), we have: Since $d$ is the element of $S$ such that $\map \nu d$ is the smallest element of $\nu \sqbrk S$: Bzout's Identity is also known as Bzout's lemma, but that result is usually applied to a similar theorem on polynomials.
So this means that gcd (a, b) is the smallest possible positive integer which a solution exists. Introduction.
Bzout's Identity is also known as Bzout's lemma, but that result is usually applied to a similar theorem on polynomials.
Sign up, Existing user? d
Bezout's identity states that for some $a,b$ there always exists $m,n$ such that $am + bn = \gcd (a, b)$ Bill Dubuque about 3 years It's not clear what you are asking, Maybe a specific example would help to clarify, Gerry Myerson about 3 years
Let $\struct {D, +, \times}$ be a Euclidean domain whose zero is $0$ and whose unity is $1$. Note: Work from right to left to follow the steps shown in the image below. y
Zum berziehen eine gewrzte Mehl-Backpulver-Mischung dazugeben.
Thus, the Bezout's Identity for a=237 and b=13 is 1 = -4(237) + 73(13).
New user?
The. Since \(1\) is the only integer dividing the left hand side, this implies \(\gcd(ab, c) = 1\). 1 is an integer and r1 is a remainder.
For any integers c,m we can nd integers ,such that gcd(c,m)= c+m.
Then, In particular, this shows that for \(p\) prime and any integer \(1 \leq a \leq p-1\), there exists an integer \(x\) such that \(ax \equiv 1 \pmod{n}\). \newcommand{\glog}[3]{\log_{#1}^{#3}#2} WebOne does not need the extended Euclidean algorithm to derive the Bezout identity: the identity can be proved in other ways.
}\). Let's see how we can use the ideas above.
,
Bezout's identity states that for some a, b there always exists m, n such that a m + b n = gcd ( a, b) How should I show the inverse mod as a modular equivalence? WHEN DOING SUBSTITUTION BE VERY CAREFUL OF THE POSITIVES AND NEGATIVES.
Lies weiter, um zu erfahren, wie du se.
a &= b x_1 + r_1, && 0 < r_1 < \lvert b \rvert \\ As an example, the greatest common divisor of 15 and 69 is 3, and 3 can be written as a combination of 15 and 69 as 3 = 15 (9) + 69 2, with Bzout coefficients 9 and 2. [
Degree of an intersection on an algebraic group3.
| x
Prove that there is a bijection g : A + B. and Without loss of generality, suppose specifically that $b \ne 0$.
b.
Web; . Since we have a remainder of 0, we know that the divisor is our GCD.
Relating two numbers and their greatest common divisor, This article is about Bzout's theorem in arithmetic.
So if we expect gcd(a,b) to equalone such xa+yb, it must be the least possible. WebBEZOUT THEOREM One of the most fundamental results about the degrees of polynomial surfaces is the Bezout theorem, which bounds the size of the intersection of polynomial surfaces.
Chicken Wings bestellen Sie am besten bei Ihrem Metzger des Vertrauens.
Since S is a nonempty set of positive integers, it has a minimum element
Idealerweise sollte das KFC Chicken eine Kerntemperatur von ca. \end{equation*}, \begin{equation*} Would spinning bush planes' tundra tires in flight be useful? The extended Euclidean algorithm always produces one of these two minimal pairs. By hypothesis, a = kd and b = ld for some k;l 2Z. Proof.
Sie knnen etwas geriebenen Parmesan beigeben oder getrocknete Kruter.
The GCD = 1 indicates that the numbers are relatively prime. \newcommand{\R}{\mathbb{R}}
Claim 1. GCD (237,13) = 1 = first non zero remainder.
Webtions, or, The Extended Euclidean Algorithm, or, Bezouts Identity.
Log in here. \newcommand{\Tf}{\mathtt{f}} Introduction. We have \(\gcd(10,3)=\).
/
For integers a and b, let d be the greatest common divisor, d = GCD (a, b).
It is an integral domain in which the sum of two principal ideals is again a principal ideal.
Is the number 2.3 even or odd?
Now, as illustrated in the example above, we can use the second to last equation to solve for \(r_{n+1}\) as a combination of \(r_n\) and \(r_{n-1}\). If you do not believe that this proof is worthy of being a Featured Proof, please state your reasons on the talk page.
If pjab, then pja or pjb. d
Web(6)Complete the following proof of Euclids Lemma: Let p be a prime, a;b 2Z. The set S is nonempty since it contains either a or a (with
}\), \((s\cdot a)+(t\cdot b) =\gcd(a,b)\text{. b \newcommand{\fmod}{\bmod}
}\), \((s\cdot 28)+(t\cdot 12)=\gcd(28,12)=4\), \(q := a\fdiv b = 28 \fdiv 12 = 2\text{.
=
tienne Bzout's contribution was to prove a more general result, for polynomials. q Use the Euclidean Algorithm to determine the GCD, then work backwards using substitution.
We find the greatest common divisor of 63 and 14 using the Euclidean Algorithm.
Wenn Sie als Nachtisch oder auch als Hauptgericht gerne Ses essen, werden Sie auch gefllte Kle mit Pflaumen oder anderem Obst kennen. D-property for Ramanujan functionsChapter 11. , by the well-ordering principle.
Some sources omit the accent off the name: Bezout's identity (or Bezout's lemma ), which may be a mistake.
Introduction2.
d Help me understand this report Cited by 2 publication s ( 3 citation statement s) References 5 publication s
However, note that as $\gcd \set {a, b}$ also divides $a$ and $b$ (by definition), we have: Consider the Euclidean algorithm in action: First it will be established that there exist $x_i, y_i \in \Z$ such that: When $i = 2$, let $x_2 = -q_2, y_2 = 1 + q_1 q_2$.
Bzout's Identity on Principal Ideal Domain, Common Divisor Divides Integer Combination, review this list, and make any necessary corrections, https://proofwiki.org/w/index.php?title=Bzout%27s_Identity&oldid=591679, $\mathsf{Pr} \infty \mathsf{fWiki}$ $\LaTeX$ commands, Creative Commons Attribution-ShareAlike License, \(\ds \size a = 1 \times a + 0 \times b\), \(\ds \size a = \paren {-1} \times a + 0 \times b\), \(\ds \size b = 0 \times a + 1 \times b\), \(\ds \size b = 0 \times a + \paren {-1} \times b\), \(\ds \paren {m a + n b} - q \paren {u a + v b}\), \(\ds \paren {m - q u} a + \paren {n - q v} b\), \(\ds \paren {r \in S} \land \paren {r < d}\), \(\ds \paren {m_1 + m_2} a + \paren {n_1 + n_2} b\), \(\ds \paren {c m_1} a + \paren {c n_1} b\), \(\ds x_1 \divides a \land x_1 \divides b\), \(\ds \size {x_1} \le \size {x_0} = x_0\), This page was last modified on 15 September 2022, at 07:05 and is 2,615 bytes. Let $\gcd \set {a, b}$ be the greatest common divisor of $a$ and $b$.
Which one of these flaps is used on take off and land?
Although it is easy to see that the greatest common divisor of 5 and 2 is 1, we need some of the intermediate result from the Euclidean algorithm to find \(s\) and \(t\text{. {\displaystyle ax+by=d.} {\displaystyle 0
Work the Euclidean Division Algorithm backwards.
It is somewhat hard to guess that \( x = -1723, y = 863 \) would be a solution. If \(a, b\) and \(c\) are integers such that \(a | bc\) and \(\gcd (a, b) = 1\), then \(a | c\). u \newcommand{\Tl}{\mathtt{l}}
such that $\gcd \set {a, b}$ is the element of $D$ such that: Let $\struct {D, +, \circ}$ be a principal ideal domain. 26 & = 2 \times 12 & + 2 \\ I understand the EA but don't know how to incorporate induction on the number of steps that EA terminates even for the base case.
Then: x, y Z: ax + by = gcd {a, b}
So the localization of a Bzout domain at a prime ideal is a valuation domain.
+ This simple-looking theorem can be used to prove a variety of basic results in number theory, like the existence of inverses modulo a prime number. A special.
Let $\nu: D \setminus \set 0 \to \N$ be the Euclidean valuation on $D$.
WebNo preliminaries such as intersection numbers, Bzout's theorem, projective geometry, divisors, or Riemann Roch are required.
a \newcommand{\checkme}[1]{{\color{green}CHECK ME: #1}} Since a Bzout domain is a GCD domain, it follows immediately that (3), (4) and (5) are equivalent.
= Since \(r_{n+1}\) is the last nonzero remainder in the division process, it is the greatest common divisor of \(a\) and \(b\), which proves Bzout's identity.
By induction hypothesis, we have: Suppose we want to solve 3x 6 (mod 2). For all natural numbers \(a\) and \(b\) there exist integers \(s\) and \(t\) with \((s\cdot a)+(t\cdot b)=\gcd(a,b)\text{.}\).